|
|
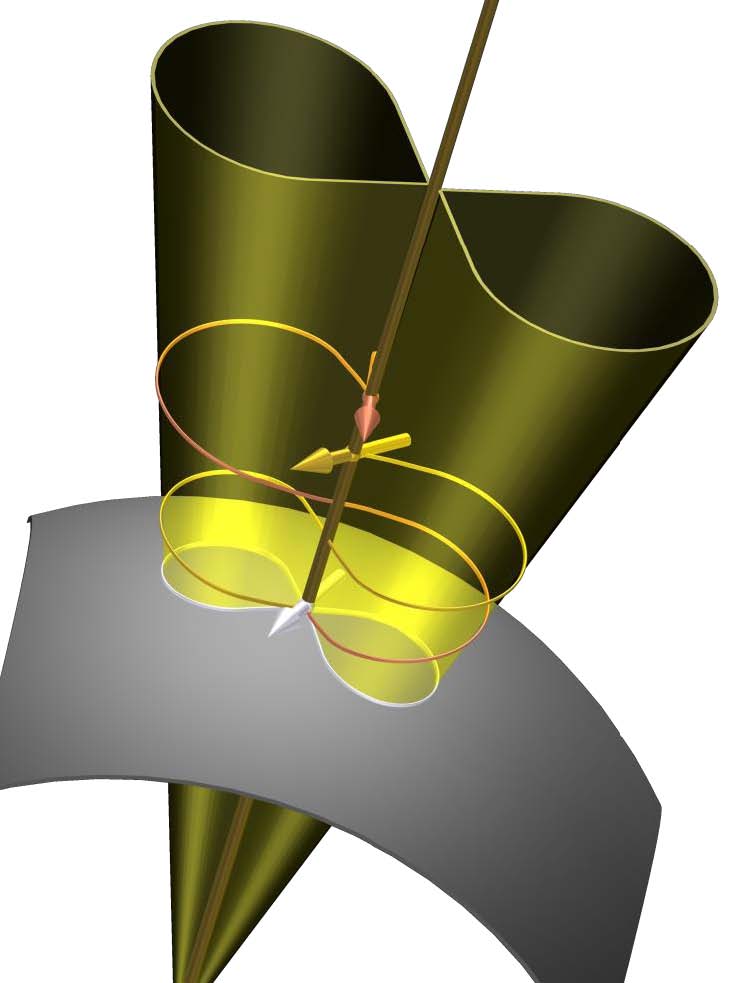
| Figure 1: Illustration of non-Abelian geometric gates in terms of fiber bundles. Depending on which loop of the path in Hilbert space is traversed first, the final state vectors corresponding to the quantum states of the system point in different directions. (large view) |
The tremendous experimental progress in the field of quantum information processing has motivated a continued quest for robust quantum operations which are reliable enough for implementing advanced quantum computation protocols. One way to protect quantum operations against detrimental influences makes use of geometry: Quantum systems can be steered through Hilbert space, such that the final state depends only on the path taken, but not on the time of the evolution. In the simplest case, the system acquires a geometric phase. However, if the system has degenerate energy levels, then matrix-valued geometric state transformations, known as non-Abelian holonomies can be obtained.
In our experiments we have observed Berry's adiabatic geometric phase of a qubit [1] and of an harmonic oscillator [2] realized as superconducting electronic circuits. We have demonstrated the noise-resilience of the adiabatic geometric phase in the limit of long evolution times, see S. Filipp, et al., Phys. Rev. Lett. 102, 030404 (2009) and [3]. Moreover, we have realized single-qubit gates based on non-Abelian holonomies by applying a two-tone microwave drive to three of the energy levels of a transmon device [4].
The main goal of our current project is to implement geometric two-qubit operations based on geometric gates to experimentally realize holonomic quantum computation, see P. Zanardi & M. Rasetti, Phys. Lett. A 264, 94 (1999), in the circuit QED architecture. We explore the sensitivity of geometric gates to external perturbations and compare their performance to quantum algorithms based on conventional gates. In this project we also explore geometric phases emerging only from vacuum fluctuations, see I. Fuentes-Guridi, et al., Phys. Rev. Lett. 89, 220404 (2002).
References:
[1] Observation of Berry's Phase in a Solid-State Qubit, Science 318, 1889 (2007)
[2] Geometric Phase and Nonadiabatic Effects in an Electronic Harmonic Oscillator, Physical Review Letters 108, 170401 (2012), also in arXiv:1109.1157
[3] Exploring the effect of noise on the Berry phase, Physical Review A 87, 060303(R) (2013), also in arXiv:1302.3305
[4] Experimental Realization of Non-Abelian Non-Adiabatic Geometric Gates, Nature 496, 482 (2013), also in arXiv:1304.5186