|
|
|
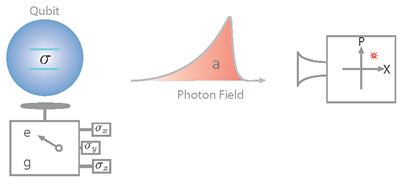
Figure 1: Sketch of the measurement scheme. |
Propagating photons are ideal carriers for distributing entanglement between distant matter systems in a quantum network. Entanglement between photons and stationary qubits has thus far been exclusively studied at optical frequencies with single atoms or electron spins. However, rapid progress in the development of superconducting circuit based quantum technologies also renders propagating microwave photons an attractive quantum information carrier. A major obstacle in measuring quantum correlations between superconducting qubits and itinerant radiation has thus far been the limited detection efficiency at microwave frequencies. In our experiments we have overcome this problem by using a quantum limited amplifier, which significantly improves the signal-to-noise ratio. In combination with novel tomography methods [1], this allows us to measure quantum correlations between itinerant microwave radiation and a stationary qubit with high fidelity [2].
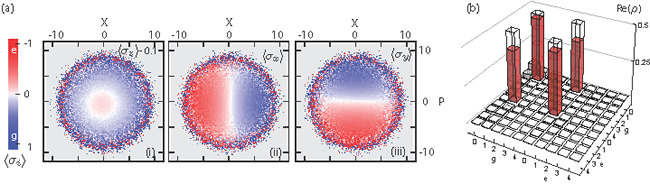
In our experiments, we use the controlled interaction between a superconducting qubit and a microwave radiation field to create entangled Bell states of the form |g1⟩+|e0⟩. Here, g and e label ground and excited state of the qubit and 0, 1, ... the photon numbers of the propagating field. We resolve the entanglement by measuring correlations between the qubit Bloch vector components ⟨σi⟩ and the quadrature components X and P of the photon field, see Fig. 1. In the σz basis, we find a higher probability of observing the qubit in its ground state at large measured field amplitudes [blue region in Fig. 2(a)(i)] which demonstrates that a photon emission is associated with the qubit to be detected in the ground state. We distinguish the quantum superposition state from a classically correlated state by analyzing the equatorial Bloch vector components ⟨σx⟩ and ⟨σy⟩ vs. X and P [Fig. 2(a)(ii)-(iii)]. Our measurement record clearly shows the expected phase dependence between the qubit and the field. Based on these measured correlations and the photon field statistics we reconstruct the full density matrix ρ of the joint system with high fidelity F = 0.83 compared to the ideal Bell state [Fig. 2(b)]. The absence of any higher photon number state populations verifies that we have entangled the qubit with no more than a single microwave photon.
 |
|
Figure 2: (a) Bloch vector components ⟨σi⟩ of the qubit conditioned on the photon field quadrature measurements X and P. (b) Real part of the measured (colored bars) and ideal (wireframes) density matrix ρ |
Phys. Rev. A 86, 032106 (2012)
http://pra.aps.org/abstract/PRA/v86/i3/e032106, also in arXiv:1206.3405v2