Researchers in many fields of physics have envisaged coupling a single quantum two-level system coherently to a quantum harmonic oscillator, see R. J. Schoelkopf & S. M. Girvin, Nature 451, 664-669 (2008)and references therein. Until 2004 this feat had only been achieved in the realm of atomic physics and quantum optics, where a single atom was observed to coherently exchange a single photon with a single mode radiation field in atomic cavity QED experiments.
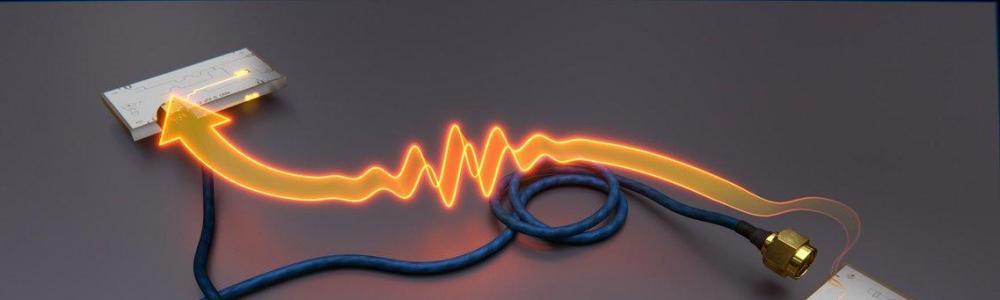
The investigation of the quantum properties of propagating microwave fields was believed to be limited by the lack of single photon detectors at these frequencies. In a ground-breaking set of experiments we have demonstrated the detection of single photon anti-bunching and sub-poissonian photon statistics in both pulsed [1] and continuous [2] microwave frequency single photon sources (Fig. 1).
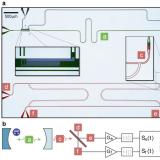
We have also shown that detection schemes based on linear amplification and quadrature amplitude detection enable the full reconstruction of the quantum state of propagating single photons [3] even in the presence of noise or limited detection efficiency. These insights have already enabled the generation and detection of entanglement between propagating photons and localized qubits, and the observation of the coalescence of indistinguishable photons at a beam-splitter, known as the Hong-Ou-Mandel effect, at microwave frequencies [4].

The circuit QED architecture [1] is extremely attractive for quantum computation using superconducting electronic circuits. It provides an ideal setting to control the coupling of qubits to their environment while at the same time allowing for the strong and controlled coupling to photons, to both control and read-out the qubit state. The off-resonant strong coupling between the qubit and the resonator can be used to perform a quantum non-demolition (QND) dispersive read-out of the qubit state.

Combining the long coherence times available in microscopic quantum systems with the strong interactions and integration available in solid state systems is a major goal in quantum information science. Atoms and ions for example can be effectively isolated from their environment allowing for coherence times of up to minutes. On the other hand, the interactions of these systems with control fields are usually weak, limiting the effective time scales over which the quantum systems can be manipulated. In a solid state environment the situation is the opposite.
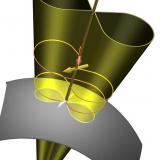
The tremendous experimental progress in the field of quantum information processing has motivated a continued quest for robust quantum operations which are reliable enough for implementing advanced quantum computation protocols. One way to protect quantum operations against detrimental influences makes use of geometry: Quantum systems can be steered through Hilbert space, such that the final state depends only on the path taken, but not on the time of the evolution. In the simplest case, the system acquires a geometric phase. However, if the system has degenerate energy levels, then matrix-valued geometric state transformations, known as non-Abelian holonomies can be obtained.